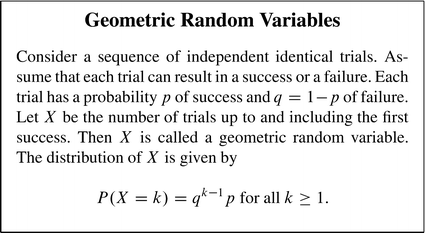
is a random variable with a .” The parameter is The geometric pdf tells us the probability that the first occurrence of success requires x number of failure independent trials, each with probability Citation/Attribution
Probability with geometric random variables — Krista King Math | Online math help
Jan 8, 2024The formula for the mean for the random variable defined as number of failures until first success is \(\mu=\frac1p=\frac10.02=50\) See Example \(\PageIndex9\) for an example where the geometric random variable is defined as number of trials until first success. The expected value of this formula for the geometric will be different
Source Image: link.springer.com
Download Image
11.2 11.2 Key Properties of a Geometric Random Variable On this page, we state and then prove four properties of a geometric random variable. In order to prove the properties, we need to recall the sum of the geometric series. So, we may as well get that out of the way first. Recall The sum of a geometric series is:

Source Image: en.wikipedia.org
Download Image
Section 6.3 Binomial and Geometric Random Variables – ppt download Apr 24, 2022We will refer to this function as the right distribution function of T. Of course both functions completely determine the distribution of T. Suppose again that N has the geometric distribution on N + with success parameter p ∈ (0, 1]. N has right distribution function G given by G(n) = (1 − p)n for n ∈ N.

Source Image: www.majorica.com
Download Image
Which Of The Following Random Variables Is Geometric
Apr 24, 2022We will refer to this function as the right distribution function of T. Of course both functions completely determine the distribution of T. Suppose again that N has the geometric distribution on N + with success parameter p ∈ (0, 1]. N has right distribution function G given by G(n) = (1 − p)n for n ∈ N. Notation for the Geometric: G = G = Geometric Probability Distribution Function. X ∼ G(p) X ∼ G ( p) Read this as ” X X is a random variable with a geometric distribution.” The parameter is p p; p = p = the probability of a success for each trial. Example 4.5.4 4.5. 4.
Algaida geometric bracelet with black Murano glass
(August 2022) ( Learn how and when to remove this template message) In probability theory and statistics, the geometric distribution is either one of two discrete probability distributions : The probability distribution of the number of Bernoulli trials needed to get one success, supported on the set ; The probability distribution of the number Detecting image similarity using Spark, LSH and TensorFlow | by Pinterest Engineering | Pinterest Engineering Blog | Medium
Source Image: medium.com
Download Image
Solved Let X and Y be independent random variables and both | Chegg.com (August 2022) ( Learn how and when to remove this template message) In probability theory and statistics, the geometric distribution is either one of two discrete probability distributions : The probability distribution of the number of Bernoulli trials needed to get one success, supported on the set ; The probability distribution of the number

Source Image: www.chegg.com
Download Image
Probability with geometric random variables — Krista King Math | Online math help is a random variable with a .” The parameter is The geometric pdf tells us the probability that the first occurrence of success requires x number of failure independent trials, each with probability Citation/Attribution
Source Image: www.kristakingmath.com
Download Image
Section 6.3 Binomial and Geometric Random Variables – ppt download 11.2 11.2 Key Properties of a Geometric Random Variable On this page, we state and then prove four properties of a geometric random variable. In order to prove the properties, we need to recall the sum of the geometric series. So, we may as well get that out of the way first. Recall The sum of a geometric series is:

Source Image: slideplayer.com
Download Image
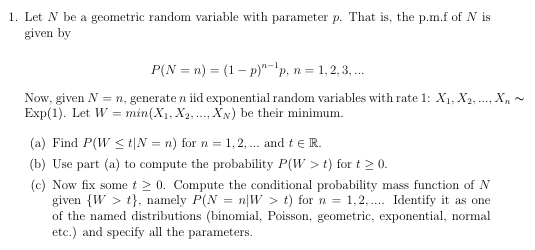
Solved 1. Let N be a geometric random variable with | Chegg.com Each of the following is an example of a random variable with the geometric distribution. Toss a fair coin until the first heads occurs. In this case, a “success” is getting a heads (“failure” is getting tails) and so the parameter \(p = P(h) = 0.5\). Buy lottery tickets until getting the first win.
Source Image: www.chegg.com
Download Image
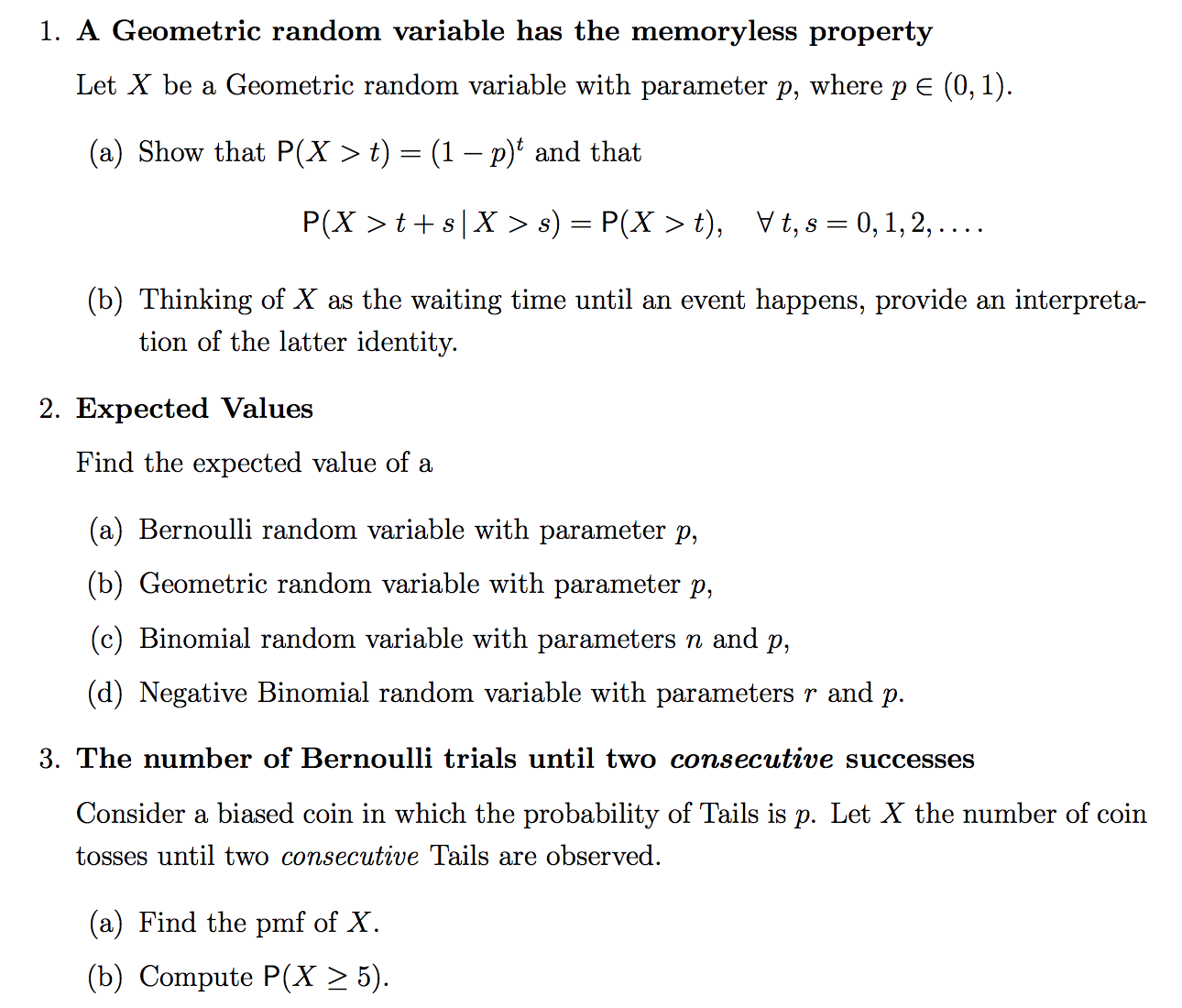
Solved A Geometric random variable has the memory less | Chegg.com Apr 24, 2022We will refer to this function as the right distribution function of T. Of course both functions completely determine the distribution of T. Suppose again that N has the geometric distribution on N + with success parameter p ∈ (0, 1]. N has right distribution function G given by G(n) = (1 − p)n for n ∈ N.
Source Image: www.chegg.com
Download Image
Let M = min (X, Y), where X and Y are independent geometric random variables with parameters p1 and p2. What is the distribution of M? – Quora Notation for the Geometric: G = G = Geometric Probability Distribution Function. X ∼ G(p) X ∼ G ( p) Read this as ” X X is a random variable with a geometric distribution.” The parameter is p p; p = p = the probability of a success for each trial. Example 4.5.4 4.5. 4.
Source Image: www.quora.com
Download Image
Solved Let X and Y be independent random variables and both | Chegg.com
Let M = min (X, Y), where X and Y are independent geometric random variables with parameters p1 and p2. What is the distribution of M? – Quora Jan 8, 2024The formula for the mean for the random variable defined as number of failures until first success is \(\mu=\frac1p=\frac10.02=50\) See Example \(\PageIndex9\) for an example where the geometric random variable is defined as number of trials until first success. The expected value of this formula for the geometric will be different
Section 6.3 Binomial and Geometric Random Variables – ppt download Solved A Geometric random variable has the memory less | Chegg.com Each of the following is an example of a random variable with the geometric distribution. Toss a fair coin until the first heads occurs. In this case, a “success” is getting a heads (“failure” is getting tails) and so the parameter \(p = P(h) = 0.5\). Buy lottery tickets until getting the first win.